


Next: The Geometric analogy
Up: The Symmetry Principles
Previous: General Theory of Relativity:
Gauge invariance of Electromagnetism:
In the 1920's Hermann Weyl pointed out a symmetry of Electromagnetism that was
different in technical detail but very similar in spirit to the Principle of
General Covariance. This symmetry he called the Gauge Principle.
Suppose we write the Maxwell Equations, not in terms of
Eand
B fields, but in terms of the four-vector potential field
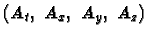 .
It can then be shown that
Maxwell's equations are unchanged if we choose a different vector field
.
It can then be shown that
Maxwell's equations are unchanged if we choose a different vector field
 such that
such that
etc., where f is a function of space as well as time, t. This
is like the triangle we had in sec. 2.2. We need an equilateral
triangle, but somehow the Physics does not depend on which corner is which.
Here we need a four vector field but if the components are all changed in the
specific form given above, it does not affect the Physics. It is important to
note that the change is not at all like a Lorentz transformation of inertial
reference axes. We have an independent change in components at each point in
space-time. This is because f is a function of space and time.
Now this situation is quite similar to the case of General Covariance, where
invariance under arbitrary coordinate changes amounted to independent Lorentz
transformations at each point. Furthermore, as in the case of General Covariance
this invariance of Electromagnetism also helps to fix the interaction
of electromagnetic fields with charged matter. We discuss this next. In Quantum
Mechanics, matter is described by a wavefunction  .
We now insist
on making the change
.
We now insist
on making the change
with the same f as in the preceding equation. Thus the phase of the wavefunction
is simultaneously changed in a space-time dependent manner. The law of interaction
of charges and electromagnetic fields is invariant under this simultaneous change.
This Principle has now been established to a great level of accuracy. It also
reproduces correctly the interaction of classical (macroscopic) currents and
charges with electromagnetic fields.



Next: The Geometric analogy
Up: The Symmetry Principles
Previous: General Theory of Relativity:
U. A. Yajnik
2001-03-14
![]() .
It can then be shown that
Maxwell's equations are unchanged if we choose a different vector field
.
It can then be shown that
Maxwell's equations are unchanged if we choose a different vector field
![]() such that
such that 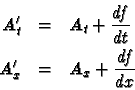
![]() .
We now insist
on making the change
.
We now insist
on making the change